
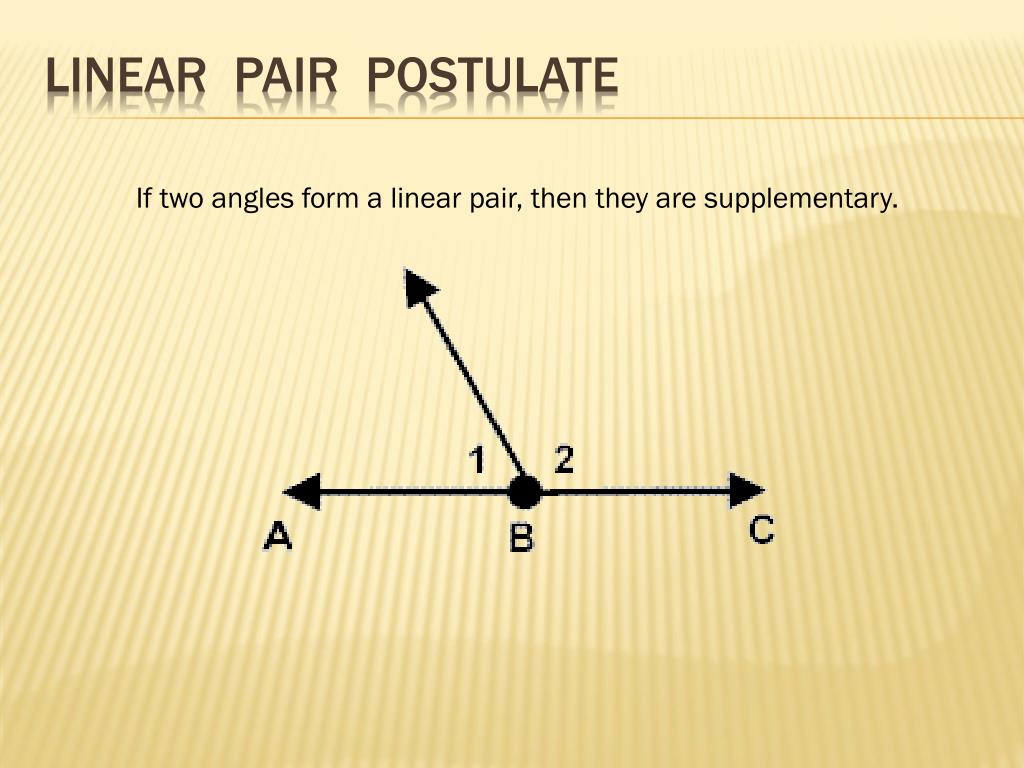
Find the other angle measures in the diagram. Identify all pairs of congruent angles in the diagram. Congruent angles in a diagram are marked by matching arcs at the vertices. m T = 121°, m P = 84° ANSWER Congruent Angles Two angles are congruent if they have the same measure. ANSWER 60°, 30° for Example 3 GUIDED PRACTICEĪNSWER T and S, P and R. Given that EFGis a right angle, find mEFH andm HFG. ANSWER 125°, 55° for Example 3 GUIDED PRACTICEĤ. Given that KLMis a straight angle, find mKLN andm NLM. ANSWER Animated Solution EXAMPLE 3 Find angle measuresįind the indicated angle measures. O ALGEBRAGiven that m LKN =145, find m LKM andm MKN. MÐBQC = x – 7 mÐCQD = 2x – 1 mÐBQD = 2x + 34 Find x, mÐBQC, mÐCQD, mÐBQD. mÐRSV = x + 5 mÐRSV = 18 + 5 = 23 mÐVST = 3x - 9 mÐVST = 3(18) – 9 = 45 Check: mÐRSV + mÐVST = mÐRST 23+ 45 =68 S T Set up an equation using the Angle Addition Postulate. Algebra Connection Slide 4 R V Extension: Now that you know x = 18, find mÐRSV and mÐVST.
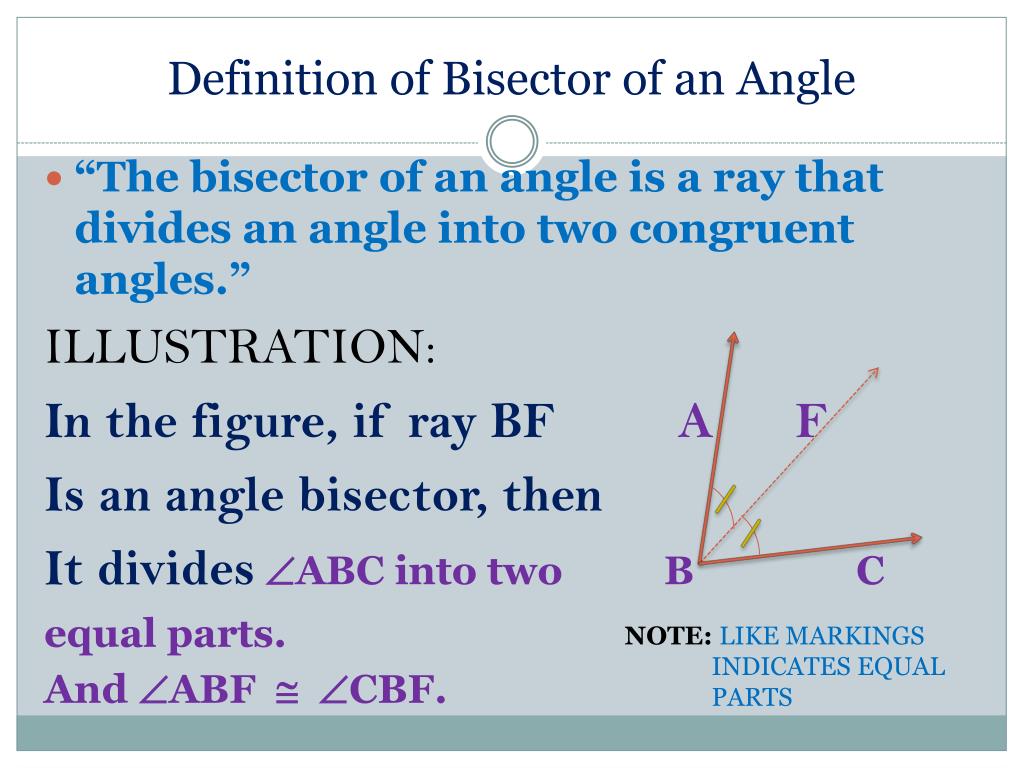
Given: mÐRSV = x + 5 mÐVST = 3x - 9 mÐRST = 68 Find x. The Angle Addition Postulate tells us: mÐGHK + mÐKHJ = mÐGHJ 95 + mÐKHJ = 114 mÐKHJ = 19. mÐABD + mÐDBC = mÐABC mÐABD + mÐDBC = 180 So, if mÐABD = 134, then mÐDBC = _ H J Given: mÐGHK = 95 mÐGHJ = 114. ÐABC is a straight angle, therefore mÐABC = 180. B A mÐAOC = 115° 50° C 65° Oĭ Example 1: Example 2: Slide 3 G 114° K 134° 46° A B C 95° 19° This is a special example, because the two adjacent angles together create a straight angle. Īngle Addition Postulate Slide 2 If B lies on the interior of ÐAOC, then mÐAOB + mÐBOC = mÐAOC. Set up the Segment Addition/Congruence Postulate.2x 4x + 6 R P Q PQ = 2x QR = 4x + 6 PR = 60 Use the Segment Addition Postulate find the measure of PQ and QR. If PQ +QR = PR, then Q is between P and R. Postulate 2-2 Segment Addition Postulate If Q is between P and R, then PQ + QR = PR. It allows us to add the measures of adjacent angles together to find the measure of a bigger angle… J K L The Angle Addition Postulate is very similar, yet applies to angles. For example: JK + KL = JL If you know that JK = 7 and KL = 4, then you can conclude that JL = 11. We discussed the Segment Addition Postulate, which stated that we could add the lengths of adjacent segments together to get the length of an entire segment. Find the measure of an angle by using definition of Angle Bisector.Īngle Addition Postulate First, let’s recall some previous information from last week….Find the measure of an angle by using Angle Addition Postulate.Sec 3.3 Angle Addition Postulate & Angle Bisector


 0 kommentar(er)
0 kommentar(er)
